EVERYTHING’S RELATIVE
Let’s say you’re sitting in a train that has just arrived at the station. As you gaze out the window, the train starts moving again even though all the passengers haven’t yet had a chance to get off. Then you realize that it isn’t the train you’re on that’s moving, but the one on the tracks beside you. This is just one of many perceptual illusions we encounter in daily life. Every day, our brain is confronted with hundreds of sensory stimuli that it has to process and make sense of. We perceive many of these stimuli through our eyes: colors, shapes, light and dark.
We interpret our environment as static while the objects in it move. This perception has been burned into human brain circuits over millennia. If something unfamiliar is added to the scene, our mind attempts to compare it to things it already knows. The conflicts that arise between the stimulus and the interpretation result in perceptual illusions. These can affect how we perceive brightness, color, size and perspective. Not only do scientists study optical illusions like these but artists have also taken advantage of them, making for fascinating photographs and paintings that lead us astray.
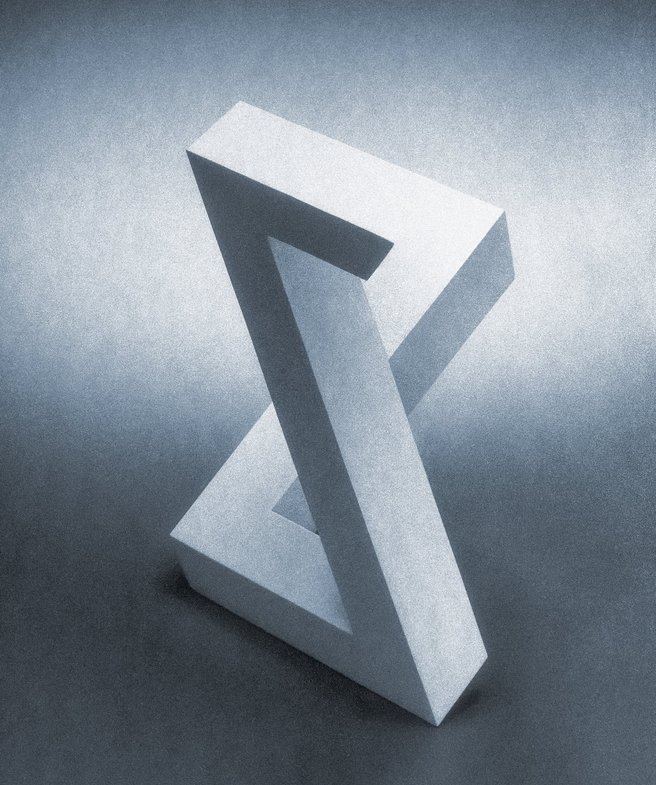
One of the most famous practitioners of this was the Dutch artist M.C. Escher, who created impossible forms and spaces in his works, not unlike the shape seen here at the left: never-ending stairwells, topsy-turvy landscapes, impossible perspectives, support elements at impossible right angles to one another. Fascinating and confusing at the same time.

In 1973, Steve Simpson, an employee of the neuropsychologist Richard L. Gregory, was walking through placid Bristol in Great Britain. Looking at the façade of a café, he discovered what seemed to be a very strange arrangement of dark green and white bricks, separated by grey lines that seemed to be running diagonally to one another. Gregory discovered that the bricks only appeared to be trapezoidal, but in actuality this was just an optical illusion. There is no uniform explanation as to why this illusion occurs. One guess is that the separating lines are seen as darker between black squares and lighter between white squares (as seen with the red lines in the picture below). Then our brain connects the lighter-colored separating lines with the corners of the white squares, the darker ones with the black squares, and that this creates the impression of slanting in our brains. In reality, however, they are simply parallel rows of black and white squares slightly offset from one another. If you pushed them so that the black and white squares alternatingly lined up with one another, you’d see a clean checkerboard pattern.

Many people are familiar with what is known as the Müller-Lyer illusion, an image with stick arrows. Discovered at the end of the nineteenth century by the psychologist Franz Müller-Lyer, the illusion is that a shaft of an arrow with heads on each end looks shorter than a shaft of the exact same length with tails on both ends (or even a shaft with a head and a tail on it). The phenomenon can be seen here at the right—both lines are the exact same length. Science doesn’t yet have a good explanation for this illusion either. It’s hypothesized that it could be a lack of coordination between the eye and brain. Others refer to Emmert’s Law, which refers to the relationship between the size of an object on the retina, its distance and the actually perceived size.
Another shift in the perception of size can be recognized in what is known as the Ponzo illusion or the “train tracks illusion,” which can be seen in the image with the train tracks. Two horizontal lines of equal length are placed on or between two parallel lines that look like train tracks. Due to the size constancy principle, the line in the rear looks longer. The reason for this is that the illustration of the train tracks is perceived spatially, so the upper bar thus appears to be located further along the tracks. If it had the same original size as the lower bar, it would logically have to appear smaller at that distant location; the brain thus concludes that it must be longer than the lower one in order to appear so large even “at a distance.”
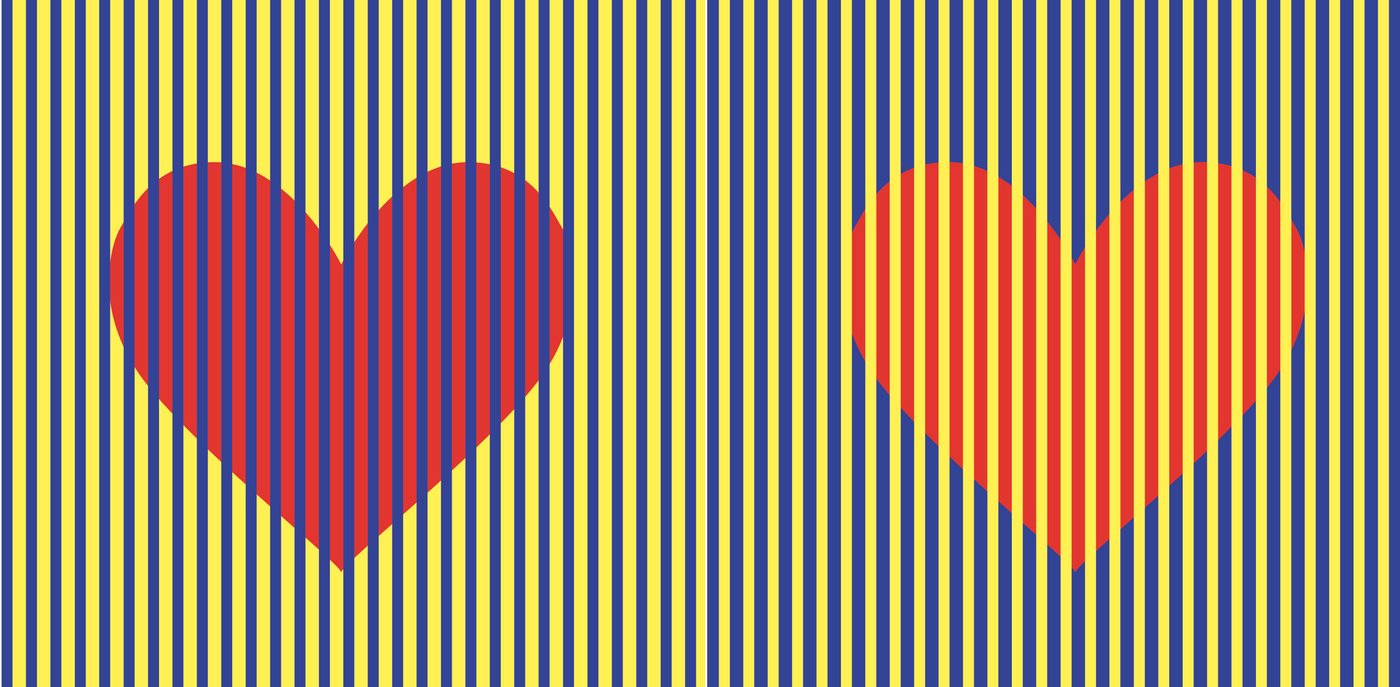
Do you remember the picture of a dress that was shared all over the internet in 2015? Some claimed the dress was blue and black, while others were absolutely certain that it was white and gold. In reality, our minds often play tricks on us when it comes to perceiving color as well as size. Take a look at the image with the two hearts in it: one is red and the other is yellow, right? Wrong! Both hearts are the exact same color of red. What has changed is that the lines running from top to bottom over the heart at the right aren’t blue, like they are on the left, but instead are yellow. It’s this shift in color that is responsible for us initially seeing the hearts as being two different colors. Our brain compares the adjacent colors with each other and then plays a trick on us: colors are always perceived in relation to their immediate surroundings. It’s called assimilation. The model for this grid is the Munker–White illusion from 1981.
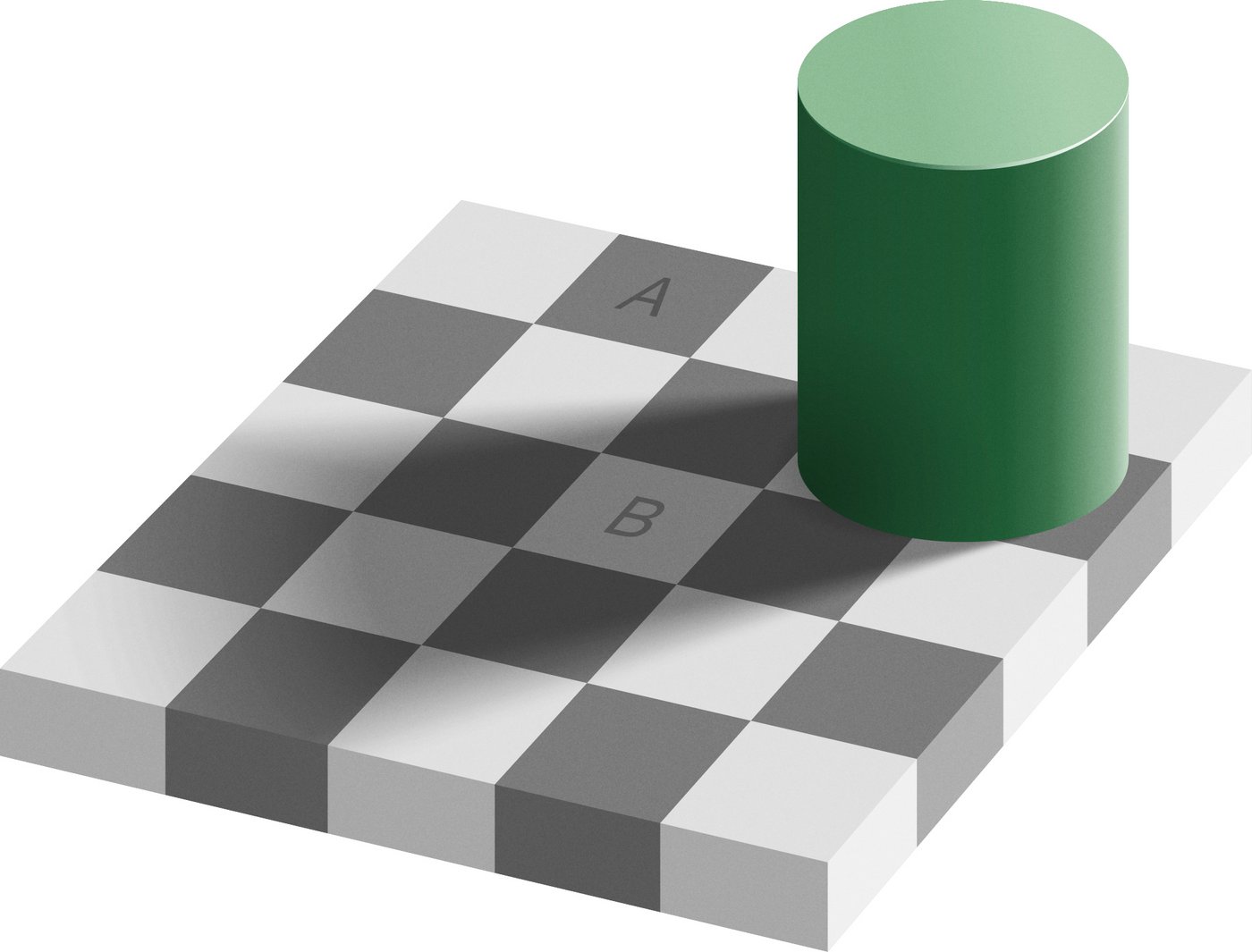
Returning to the checkerboard pattern, this time we’re less interested in the lines than we are in the relative brightness of the squares. This is perceived very subjectively by different people, and is mainly based on the experiences our minds have had perceiving light and dark. For instance, colors usually seem much brighter at twilight than they do in strong sunlight. This is why a gray line that has the exact same color tone along its entire length looks darker in lighter surroundings than it does in darker surroundings. The same is true of our experiences with light and shadow. The square marked B is located in the shadow of the green cylinder. Our brain assumes that B is lighter than A because B has been made “darker” by the shadow falling on it. In actuality, the squares marked A and B are the exact same shade of gray and have an identical brightness.